Trigonometry
If there is one idea central to trigonometry, it is that all right triangles with one of their other angles equal are similar, which means that ratios of corresponding sides are equal. For example, all right triangles with a 30° angle are similar to each other, so the ratios of the leg opposite the 30° angle to the hypotenuse are always the same (in this case 1/2). This ratio of opposite to hypotenuse is called the sine of the angle; that the sine is a function of the angle depends on the fact that all right triangles with a 30° angle (or any other angle) arem similar. Since triangles have 3 sides, there are 3P2 = 6 possible ratios of pairs of sides, leading to the 6 trig functions of the acute angles in a right triangle, the sine, cosine, tangent and their reciprocals. The mnemonic SOH CAH TOA can help us remember the first three.
Trig ratios on the unit circle
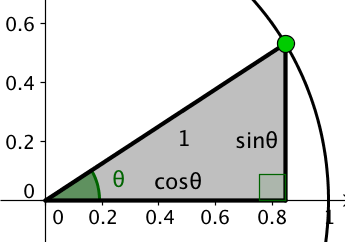
We can extend trigonometry beyond mere right triangles with the unit circle, a circle of radius 1, centered on the origin. If you want to visualize the trig functions of an acute angle θ, create an angle with the vertex of the angle at the origin, the initial ray of the angle along the positive x-axis, and the terminal ray at an angle of θ counterclockwise from the initial ray. Make a triangle by connecting the origin, the point where the second ray intersects the circle, and the projection of that point on the x-axis. This triangle is said to be in standard position, not because there's anything special about it, but rather it's just a convention to make it easier to talk about this stuff.
Here is the first mental leap toward unit circle trig: In SOH CAH TOA trig, the trig functions are thought of as ratios, so for example the cosine is adjacent/hypotenuse, but in this picture the cosine is pictured as just the adjacent. That's part of the beauty of the unit circle: in this triangle, the hypotenuse is 1 so
adjacent/hypotenuse = adjacent/1 = adjacent
If θ is in the first quadrant, cosθ and sinθ, the lengths of the legs of the right triangle, are also the x- and y-coordinates of the point where the ray of the angle intersects the circle. The big idea of the unit circle is that we switch our perspective and define the cosine and sine of θ to be the coordinates of that point. This allows us to take the cosine and sine of any angle, even though angles above 90° cannot be in any right triangle.
In the applet, uncheck tan/sec and cot/csc. Convince yourself that all the labels are correct, both the sides labeled cosθ and sinθ and the coordinates of the point. The black triangle is called the reference triangle because it is used for reference to help find the trig functions of θ. Now experiment with angles 90° and greater and 0°. Notice that there is no triangle with those angles, but the green point still has coordinates, so cosθ and sinθ still make sense. The reference triangle now shows the reference angle ρ. Determine the relationship of the reference angle ρ and the original angle θ in each quadrant. Convince yourself that the cosine and sine of θ differ from those of ρ only by their signs. Thus you can still use the reference triangle to determine the trig functions of θ
Less familiar are the two triangles similar to this one. which each have one side of length 1, which help visualize the other basic trig functions: tangent, secant, cotangent and cosecant.
Uncheck tan/sec and/or cot/csc and see if you can keep in mind:
- The definitions of these other functions in terms of the cosine and sine (e.g. tanθ = sinθ/cosθ)
- Similar triangles have corresponding sides in proportion and
- One of the sides of each triangle is a radius, so it has length 1.
So for example, to see why the opposite leg in the red triangle is labeled tanθ, notice that the adjacent leg of the red triangle has length 1. So in the red triangle, opposite/adjacent = opposite/1 = opposite. But since that triangle is similar to the black triangle, that ratio should also equal the black opposite/adjacent ratio, which is sinθ/cosθ = tanθ. The other sides of the red and blue triangles can be discovered similarly.
Can you see:
- Why the sides labeled secθ, cscθ, and cotθ deserve those labels (see the justification of tanθ above);
- Where the names tangent, cotangent, secant and cosecant come from? (Think about the geometric meanings of the words tangent, secant and complement);
- Why the three Pythagorean trig identities are true?
From unit circle to function graph
Here's another mental leap. We can make an angle of any measure from the initial ray. That angle can be greater than 360° (2π radians) or negative (a clockwise angle). Thus the trig functions can be thought of as functions of ℝ (where the input is the angle in degrees or radians). Many students are familiar with the unit circle and the familiar wavy graphs of the sine or cosine functions, but wonder how they are connected.
On the unit circle, the input angle θ is a central angle in the circle and the intersected point is (cosθ, sinθ). But in a traditional graph of a function, the x-axis is the input and the y-axis is the output. That's very different! How do we reconcile those ways of looking at it?
Here is a way to visualize the relationship. In the following applet, blue represents the input θ and red represents the output sinθ. Notice how all the blue things really represent θ and how all the red things represent sinθ (though cosθ snuck in once). On the unit circle, notice the angle θ and the point (cosθ,sinθ). Now look right to the point (θ,sinθ). Notice that the points share a y-value, sinθ, but the blue input θ on the function graph corresponds to the blue angle θ in the unit circle. There is a particularly beautiful connection if we think of the angle in radians: in that case, θ is the actual arc length of the blue arc marked θ, and it is also the length of the blue segment on the function graph from the origin to the blue point labeled θ. You can imagine wrapping a string around the unit circle starting from (1,0) and ending at the point (θ,sinθ). The length of that string is the radian measure of θ. That is also the length of a string starting at the origin and extending along the x axis to θ.
passionatelycurious.com • Math tutor • Computer Science tutor • What's with the '90s Web site? • contact
